Practicing math

Welcome to math forum,

To get a little practice thinking about this kind of thing, consider
the question: do human beings have free will? Is there any outcome or
situation that can be explained by free will that can't also be
explained by predestination, and vice versa? There isn't. examples on free math; In which
case, what is the point of asking the question?

(If you don't understand what I'm getting at, find a copy of the book
_Pragmatism_ by William James and read it. In fact, you should read it
anyway. It will save you a lot of useless head-scratching over the
course of your life.) continue reading on online math forum.

Realworld math

Welcome to online math forum,

The application of math to the real world is based on induction: we
try something repeatedly and see that, yes, our calculations about
circumference do work in the real world, so the assumptions on which
they are based must be accurate.

If we measured big enough circles, we
would find that relativity makes it not quite work right; math help; that means
that the world doesn't quite match the Euclidean geometry on which our
calculations are based. But induction does show us that it is a close
enough approximation in normal cases.
Learn more on math tutors online.

Math Puzzlers

Welcome to math tutor online free,

Certain "puzzlers" in mathematical recreations defy our sense of
experience, leaving you wondering if the answer to a problem can
really be true.

One example is the well-known birthday probability problem, and the
answer that 23 people in a room leads to a 50/50 probability that two
will share the same birthday.

Another is the problem of adding, e.g., "only" one meter to a rope
around the Earth, and determining that the "gap" created between the
lengthened rope and the Earth is about 16 cm. How can it be that
adding such a short length to the rope will result in such a large
gap? Of course,examples on online math forum; it's easy to show using
simple algebra that the result
is a pure value ("amount or rope added"/2pi) independent of any
circumference, so that whether you do it around a superball or around
Jupiter the result will be the same.
Hope the above explanation was useful, now math forum.

Math encryption algorithm

Welcome to math tutor online free,
Every time you send a credit card number over the Internet, it gets
encrypted by your browser, and the encryption algorithm is based on
the theory of prime numbers. At some point, electronic money will
become as common as paper money, and _that_ will also be based on the
theory of prime numbers. examples on math forum;
And what's used more in the real world than
money?

The importance of prime numbers is that any integer can be decomposed
into a product of primes. For example, if you want to know how many
different pairs of numbers can be multiplied to get 360, you can start
trying to write them down,

1 * 360
2 * 180
3 * 120
4 * 90
5 * 72
6 * 60

checking every single number up to 180, and hope that you don't miss
any; or you can decompose 360 into its prime factors,

360 = 2 * 2 * 2 * 3 * 3 * 5

with the assurance that every factor of 360 will be a product of a
subset of these prime factors.
Learn more on online math forum.

Math Circle experiment

Welcome to math help online,

The circumference
divided by the diameter is the same for every circle' is only true if
you're talking about a 'flat' space. But here's an experiment you can
do to get a feel for what it would be like to live in a 'curved'
space:

Look at a globe, like this one:

    

Call the horizontal circle that goes through the top of South America
(i.e., the equator) 'Circle 1', and the horizontal circle that goes
through Chicago 'Circle 2'. more examples on online math tutors;
If you measure the 'radius' of each circle
along the surface of the earth from the north pole (which is the
'center' of each circle), then dividing circumference by diameter
gives you two different values of pi, doesn't it?

In fact, if the earth were perfectly spherical, the 'radius' of the
equator (measured along the surface) would be 1/4 of its circumference
(do you see why?), so the ratio of circumference to diameter - that
is, 'the value of pi' - would be exactly equal to 2, rather than
3.14...
learn more on online math forum.

Planning math

Welcome to free online math tutors,

knowing ahead of time what the teacher is going to say makes
class a _lot_ less frustrating - and quite a bit more interesting.
(It's a little like when you see a movie again - the second time
around, you already know what will turn out to be significant, math forum; so you
can notice all kinds of details that escaped you the first time
around.)

And this way, when the time for a test rolls around, you'll know that
you already _know_ all the material, so you won't _need_ to study,
except perhaps to go back through the chapter and make sure you can
still work the practice problems. continue learning on free math tutor online.

Rules of logical derivation

Welcome to math tutors online for free
The rules of logical
derivation. This is a less popular thing to do, because the legal
steps in a mathematical derivation are typically very obviously truth-
preserving. math help, Here's a simple example of a mathematical derivation:

1) Assumption: Every natural number has a successor
that is itself a natural number.
2) Assumption: Zero is a natural number.
3) Definition: One is the successor of zero.
-------------------------------------------
4) Theorem: One is a natural number.

The only claim being made here is that if we accept the two
assumptions and the definition above, then we must accept that one is
a natural number. learn more on math forum.

Learn math by counting apples

Now if I have the two apples I counted yesterday, and you give me
three more apples, I can count them again and I know there will be
five more examples on free online math help. I don't need to know anything else
about the apples to know this; addition is a property of the numbers themselves,
independent of any other properties the apples may have.

This means I can forget
about the apples themselves, and even, if I wish, forget about the
process of counting and the perception involved in doing that. Here we
have entered the realm of mathematics, Here in math help where we deal with abstract
numbers rather than specific counted items. And within this realm,
since we are no longer dealing with perception, what we say can be
exactly true - although when we take our conclusions back to the
"real" world, we will have to deal with the possibility that our
perceptions are inaccurate: me math helper counted wrong, apples can disappear
spontaneously, or whatever.

I might try to apply addition to something
for which it doesn't work (adding, say, a liter of sugar to a liter of
water, and expecting 2 liters of sugar water); then the problem is not
in the math but in the application. It is the job of science (or
merely of experience) to determine what mathematical models apply to a
given situation.

Math beyond Arithmetic

Greetings from Online math tutoring,

You might want to
gather different ideas by looking it up in various dictionaries or
encyclopedias, and reading the introductory chapters of several
popular books on math help, which may tell what the authors think math is
all about. There is no one "correct" answer.

As you can see, math goes far beyond arithmetic, or even algebra and
geometry. All sorts of logical thinking fit this description. And math
is a very creative field, involving exploration of the unknown, not
just learning rules we are told to follow. Mathematicians invent
abstract worlds, and discover all the surprises in them that are never
noticed by those who don't look for the abstractions behind the
reality.
Now let me explain what is free math tutoring online

Math beyond arithmetic

You might want to
gather different ideas by looking it up in various dictionaries or
encyclopedias, and reading the introductory chapters of several
popular books on math, which may tell what the authors think math is
all about. There is no one "correct" answer.

As you can see, math goes far beyond arithmetic, or even algebra and
geometry. All sorts of logical thinking fit this description. And math
is a very creative field, involving exploration of the unknown, not
just learning rules we are told to follow. Mathematicians invent
abstract worlds, and discover all the surprises in them that are never
noticed by those who don't look for the abstractions behind the
reality.ma

mathematics is a discipline

Welcome to math tutor online for free,
This session helps students who need help with math,

mathematics is a discipline that seeks understanding
of the patterns and structures of constructs of the human mind.
Understanding has no end to its depth, and mathematics seeks
the highest standards of understanding by demanding rigor in its
foundations and in its development. Rigor is achieved by responsible
attention to the principles of logic.

In a strict sense, mathematics differs from science, if we accept
that science is the discipline that seeks understanding of the
physical world by means of the scientific method. (The scientific
method is the procedure by which hypotheses are proposed and subjected
to experiments designed to expose weaknesses in the hypotheses.)
The reason mathematics differs from this is because mathematics does
not, in a pure sense, attempt to describe the physical world.
Mathematical theorems are not tested against nature, but against
logic. more explanation in math forum.

What is a Polygon

Let us study what is polygon and kinds of polygon,

A polygon is a two-dimensional object; it is a plane shape with straight sides. A pentagon is a regular polygon, it is defined as a pentagon which is all the five sides are congruent and all the five sides of interior angles are congruent. That is a polygon, both equiangular and equilateral. Important point is all the regular polygons are convex.

Polygon:

A polygon is a 2-dimensional object; it is a plane shape with straight sides.

Polygon is of two types,

1. Irregular polygon

2. Regular polygon

I hope the above explanation was useful, now let me give sample questions to solve Tetrahedron in a unit sphere.

Triangles Three

Let us study about fire triangle,

Triangle is one of the basic shape in geometry, and it is a closed figure with three sides. There are different types of triangles are available and You can categorized the triangle based on their sides and angles.

Three types of triangle, it is classified based on their sides and four types of triangles based on their angles.Let us discuss about three basic types of triangle; it is classified based on their sides.

Classifying triangles based on their Sides.

1) Equilateral triangle

2)Isosceles Triangle

3) Scalene Triangle

I hope the above explanation was useful, now let us study 1 gallon in litres

Introduction for analytical geometry

Here we are going to see some basic principles for solving problems in analytical geometry basics.

Coordinates in analytical geometry
Coordinate system is a system uses set of numbers and point in a XY plane to represent a geometrical shape. For example, to indicate a line in a XY plane we need two points.

Equations of lines and curves in analytical geometry
We use equations to represent lines and curves to denote them in a coordinate plane. For example, the equation of a line is given by, y = mx + b and this type of line equations are first order equations. The equations of curves given by second order equations. For example, the equation of a circle is given by, = , and the equation of a parabola is given by by = 2ax.

Distance between two points in analytical geometry
Let (x1, y1) and (x2, y2) be two points in a coordinate plane. The distance between these two points are given by, I hope the above explanation was useful.

Explain Prime Number

Let us study about prime number,

A prime number is a whole number greater than 1, whose only two whole-number factors are 1 and itself. The first few prime numbers are 2, 3, 5, 7, 11, 13, 17, 19, 23, and 29. As we proceed in the set of natural numbers N = {1, 2, 3, ...}, the primes become less and less frequent in general. However, there is no largest prime number. For every prime number p, there exists a prime number p' such that p' is greater than p. This was demonstrated in ancient times by the Greek mathematician Euclid.

I hope the above explanation was useful, now let us study Steps to divide fractions.

Explain Exponential rules

Let us study about Exponential rules,

Introduction to exponential algebra rules:

Exponential algebra rules is nothing but, it is used solve the exponents problems in algebra. Exponent of an integer that is shows you how many times the number is to be used in a multiplication. Exponent is shown as a small number to the right and over the base number. In this example: m2 = m × m (some another name for exponent is power or index). In this topic, we see about exponential algebra rules.

I hope the above explanation was useful, now let me explain about laws of logarithms.

Descending order

Let us study about Descending order.

Sometimes we place the numbers in order from highest (biggest) number to lowest (smallest) numbers. Highest to lowest, this type of ordering is called "Descending Order".

"Steep descent" is perfect example for descending order.

These two orders are the important orders in ordering numbers.

Examples problems for Ordering greatest to least:

1. Place the following numbers in greatest to least order.

6, 8, 1, 3, 7, 4, 9, 2

Solution:

Place the numbers ordering from biggest numbers to smallest number.

9, 8, 7, 6, 4, 3, 2, 1

These numbers were form as greatest to least ordering.

I hope the above explanation was useful, now let me explain about prime numbers up to 100.

Applications of Derivatives

Study about the applications of derivatives,
Let us began this chapter with the following statement:

* Often a physician may want to test how small changes in dosage can affect the body's response to a particular drug.

* An economist may want to study how investment changes with variation in interest rates.

* How the velocity of a heavy meteorite entering the earth's surface, changes with the its distance from the earth's surface.

* For a given volume of oil, what is the least expensive shape of an oil can?

These questions and many, more such practical problems can be expressed and solved by applying derivative.

Differential calculus can be considered as mathematics of motion, growth and change where there is a motion, growth, change. Whenever there is variable forces producing acceleration, differential calculus is the right mathematics to apply. Application of derivatives are used to represent and interprete the rate at which quantities change with respect to another variable. Most of the changes are considered in terms of independent variable time. But there is no restriction that the changes are considered with respect to time only, as we have seen in the above mentioned, statements.
I hope the above explanation was useful, now let me explain Trigonometric Equations.

Introduction of Cartesian coordinate system

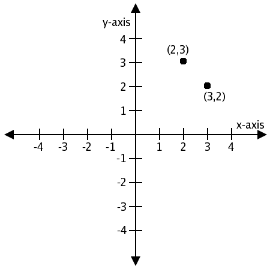Let us study about cartesian coordinate system,
The Cartesian coordinate system was developed by the mathematician Descartes during an illness. As he lay in bed sick, he saw a fly buzzing around on the ceiling, which was made of square shaped tiles. As he watched he realized that he could describe the position of the fly by the ceiling tile he was on. After this experience he developed the coordinate plane to make it easier to describe the position of objects.

one of the coordinates in a system of coordinates that locates a point on a plane or in space by its distance from two lines or three planes respectively; the two lines or the intersections of the three planes are the coordinate axes.

I hope the above explanation was useful.

A Basis for a Vector Space

Let V be a subspace of R ⁿ for some n. A collection B = { v₁, v₂, …, v _r } of vectors from V is said to be a basis for V if B is linearly independent and spans V. If either one of these criterial is not satisfied, then the collection is not a basis for V. If a collection of vectors spans V, then it contains enough vectors so that every vector in V can be written as a linear combination of those in the collection. If the collection is linearly independent, then it doesn't contain so many vectors that some become dependent on the others. Intuitively, then, a basis has just the right size: It's big enough to span the space but not so big as to be dependent.

Example 1: The collection { i, j} is a basis for R², since it spans R² and the vectors i and j are linearly independent (because neither is a multiple of the other). This is called the standard basis for R². Similarly, the set { i, j, k} is called the standard basis for R³, and, in general,
is the standard basis for R ⁿ.

I hope the above explanation was useful.